Hand Rankings Holdem

1. Hand Ranking 6+ Holdem
2. Starting Hand Ranking Holdem

As you have enlightened your knowledge about how to play Texas Hold’em poker, the five-card combination is determined to be the best based on the hand ranking.To fathom the hand ranking better we have fabricated a few frequently asked questions (FAQ) from which you can understand the hand ranking with live game scenarios. Each Poker hand in the game has a Poker hand ranking that is compared against the competitor’s rank in order to decide who is the winner. In high games like Texas Holdem and Seven-card stud the Texas Holdem winning hand emerges as the champion. A texas hold'em hand is dominated if it has 3 or fewer outs against a hand it faces, like AQ against AK. In this example only a Q can help AQ, an A will not. A hand like AK is a 3 to 1 favorite over hands it dominates like AQ, AJ, A9, KQ, KT, etc. 32 is only a 2 to1 favorite).

In this lesson you’re going to learn the first and most important step about how to play poker by learning the all important poker hand rankings.

• A standard poker hand consists of five cards.
• Each poker hand is ranked in a set order.
• The higher the rank, the less chance statistically you have of getting it.
• The higher the rank of your hand the better, because two pairs always beats one pair, and a flush always beats a straight.
• When two or more players have a hand of the same rank, then there are more ways to determine the best hand.

On this site you can find all possible combinations of preflop hands that can occur in Texas Hold'em Poker. As a bonus you will also learn the nicknames of the different hands. The hands are ranked from #1 to #169, where #1 is the best. This ranking is applicable when the poker table is full ring (9-10 people). In poker, players form sets of five playing cards, called hands, according to the rules of the game. Each hand has a rank, which is compared against the ranks of other hands participating in the showdown to decide who wins the pot. In high games, like Texas hold 'em and seven-card stud, the highest-ranking hands win.

Hopefully all these points will make perfect sense by the end of this lesson.

Poker Hands (from Best to Worst)

Be sure to pay close attention and memorize the poker hand rankings. Let’s start with the best possible hand in poker….

Royal Flush

A Royal flush consists of five cards of the same suit, in sequence from 10 through to Ace. Remember that all suits are equal in poker. If two or more players hold a royal flush (highly unlikely) then the pot is split, i.e. the players share the winnings.

Straight Flush

Five cards of the same suit, in sequence. This example shows a Jack high straight flush. If two or more players hold a straight flush then it is the highest that wins. For example, a Queen high straight flush beats a Jack high straight flush. You will notice that this is very similar to a Royal flush, and that’s because a Royal flush is in fact an ace high straight flush – but it’s given its very own ranking.

Four of a Kind

This hand contains four cards of the same rank/value. This example shows four 8’s, plus a 5 (remember that all poker hands must have five cards). If two or more players have four of a kind, then the highest value wins (e.g. four 9’s beats four 8’s). If two or more players share the same four of a kind, which can happen when using community cards (more on that later) then the winner is decided by the fifth card. So a player with four 8’s and a 6 would beat a player with four 8’s and a 5.

Full House

A full house contains three cards of the same rank, plus a pair. In our example you can see three 10’s and a pair of 7’s. The value of the three matching cards determines the strength of a full house. So three Jack’s with a pair of 7’s would beat our example hand. If players share the same three cards, which is possible when using community cards, the strength of the pair is then taken into account. So, three 10’s and a pair of 8’s would beat our example hand.

Flush

Five cards of the same suit in any order. Our example shows a Queen high flush. If two or more players have a flush then the player with the highest ranked card wins. If the players share the same high card then it’s determined by the value of the 2nd, 3rd, 4th, and 5th card respectively.

Straight

This hand contains five unsuited cards in sequence. Our example shows a King high straight. In the event of a tie, the best straight is determined by the highest ranked card. A straight consisting of 8, 9, 10, J, Q, would lose to our example hand. But a straight consisting of 10, J, Q, K, A, would win. Also note that an Ace can be used as the low card for a straight of A, 2, 3, 4, 5. This would lose to a straight of 2, 3, 4, 5, 6.

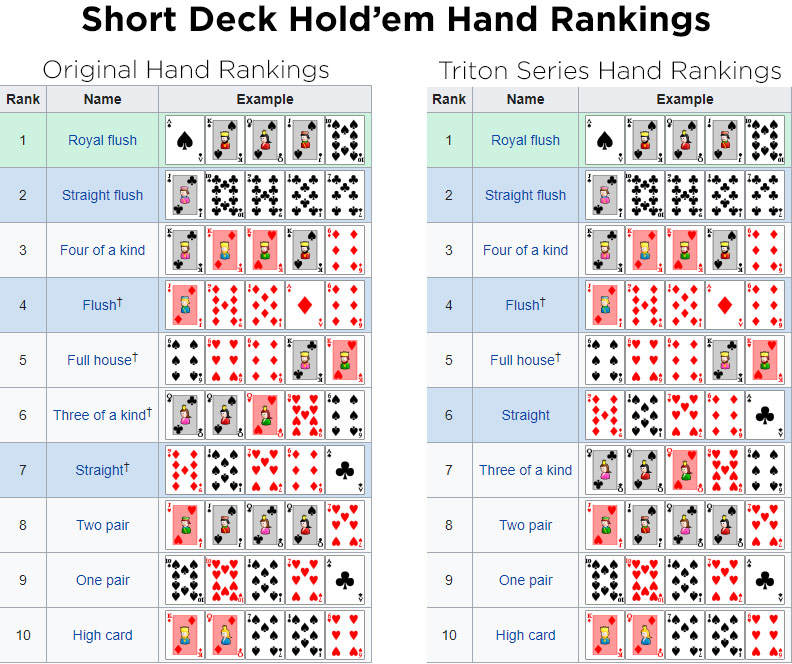
Three of a Kind

Three cards of the same rank, and two unrelated cards. Our example shows three 4’s. Three 5’s would beat our example hand, three 6’s would beat three 5’s, and so on. If players share the same three cards, then the value of the highest unrelated card would count and if necessary, the value of the second unrelated card. So, three 4’s with Jack, 8, would beat our example hand. As would three 4’s and 10, 9 (because 9 is higher than 8).

Two Pair

Two cards of matching rank, with another two cards of another rank, plus an additional card. In the event of a tie, the highest pair wins. If players share the same highest pair, then the value of the next pair wins. For example, a pair of Aces, and a pair of 6’s would beat our example hand, as would a pair of Kings and a pair of 7’s. If two or more players share the same two pair, then the value of the fifth card counts. So, a pair of Kings, a pair of 6’s, with a 4, would beat our example hand.

One Pair

A paired hand contains two cards of matching rank, plus three additional cards. The value of the pair determines who wins in the event of a tie. For example a pair of 10’s beats our example hand. If players share the same pair then the best hand is determined by the value of the highest additional card. If this is the same then it goes to the second card, and if necessary the third. So, a pair of 9’s with an Ace, 2, and 10, would beat our example hand. As would a pair of 9’s, King, 10, and a 3.

High Card

Hand Ranking 6+ Holdem

If a hand doesn’t fall into any of the above categories, then it is judged on the value of the highest ranked card among the five. In this example we have a hand which is Queen high. If players share the same highest card, then it goes to the value of the 2nd, 3rd, 4th, and even 5th card if necessary. A hand of Queen, 10, 9, 5, 4, would beat our example hand.

Community Cards

As you already know, a poker hand consists of five cards. In many variations of poker, players receive or can choose from more than five cards. For example, in Texas Hold’em each player is dealt two private cards, but can also use the five community cards that are available for all the players to use. This makes a total of seven cards, but each player must choose their best five cards to make their best possible hand. Here’s an example:

In the above example, the best five cards among total of seven (two private cards and five community cards) would be combined to make a flush.

Once you have the basic rules of poker understood, it’s time to start building a powerful strategy. See how our friends at Red Chip Poker built the perfect course to give you the perfect playbook…

Conclusion

If you don’t fully understand the poker hand rankings then please read through the list again. It’s vital that you know which hand beats which. Of course, how good a poker hand is, is very dependent on which type of poker game you are playing and other factors such as the number of other players you are playing against. You will learn the true strength of a poker hand as you gain experience of playing the game.

We have created a printable poker hand rankings chart that you can use as a source of reference. Hopefully we’ve explained the poker hand rankings to you well enough whereby you don’t need this chart, but it still might be handy for some. The chart will load as a PDF (link opens in a new window) and you’ll need to have Adobe Acrobat installed on your computer to be able to view it.

Make sure that you memorize the poker hand rankings before moving onto the next lesson.

By Tim Ryerson

Tim is from London, England and has been playing poker since the late 1990’s. He is the ‘Editor-in-Chief’ at Pokerology.com and is responsible for all the content on the website.

Starting Hand Ranking Holdem

Introduction

The following table ranks the top hands in an 8-player game. This table assumes that all players stay in until the end.

Explanation of column headings.

• Cards: Initial two-card hand.
• Probability of win: Probability that this hand will win, or tie for the win.
• Average win: This is how much the player will win on average, including his own bets, if the player does win. This is less than 8 because sometimes the player will have to split the pot.
• Expected value: This is how many units the player can expected to win (positive) or lose (negative) with this hand. For example if the player had a pair of aces and contibuted $1 to the pot then the player could expect to have a net win of $2.09.
• Probability: Probability of getting this hand to begin with.
• Additive probability: Probability of getting this hand or any stronger hand to begin with.

Initial Hold'em Hands in Rank Order for 8-Player Game

Cards	Probability of Win	Average Win	Expected Value	Probability	Additive Probability
Pair of A's	39.05%	7.94	2.099	0.45%	0.45%
Pair of K's	33.26%	7.92	1.6328	0.45%	0.9%
Pair of Q's	28.71%	7.88	1.2628	0.45%	1.36%
A/K suited	26%	7.67	0.9953	0.3%	1.66%
Pair of J's	25.13%	7.84	0.9705	0.45%	2.11%
A/Q suited	24.51%	7.6	0.8628	0.3%	2.41%
K/Q suited	23.72%	7.61	0.8043	0.3%	2.71%
A/J suited	23.41%	7.53	0.7616	0.3%	3.02%
Pair of T's	22.32%	7.79	0.7395	0.45%	3.47%
A/K unsuited	22.68%	7.61	0.7264	0.9%	4.37%
K/J suited	22.66%	7.53	0.7073	0.3%	4.68%
A/T suited	22.55%	7.45	0.6803	0.3%	4.98%
Q/J suited	22.1%	7.52	0.6622	0.3%	5.28%
K/T suited	21.87%	7.47	0.633	0.3%	5.58%
Q/T suited	21.38%	7.45	0.5936	0.3%	5.88%
J/T suited	21.26%	7.44	0.5813	0.3%	6.18%
A/Q unsuited	20.98%	7.51	0.5765	0.9%	7.09%
Pair of 9's	19.89%	7.81	0.5527	0.45%	7.54%
K/Q unsuited	20.31%	7.52	0.5281	0.9%	8.45%
A/9 suited	20.28%	7.39	0.4981	0.3%	8.75%
A/J unsuited	19.7%	7.41	0.4607	0.9%	9.65%
K/9 suited	19.5%	7.42	0.4466	0.3%	9.95%
A/8 suited	19.66%	7.32	0.4386	0.3%	10.26%
K/J unsuited	19.1%	7.43	0.4183	0.9%	11.16%
Pair of 8's	18.19%	7.79	0.4164	0.45%	11.61%
T/9 suited	19.18%	7.38	0.4162	0.3%	11.92%
Q/9 suited	19.03%	7.41	0.4107	0.3%	12.22%
J/9 suited	18.96%	7.4	0.4032	0.3%	12.52%
A/5 suited	19.29%	7.21	0.3914	0.3%	12.82%
A/7 suited	19.12%	7.26	0.3883	0.3%	13.12%
Q/J unsuited	18.67%	7.41	0.3832	0.9%	14.03%
A/T unsuited	18.74%	7.31	0.3705	0.9%	14.93%
A/4 suited	18.86%	7.23	0.3638	0.3%	15.23%
A/6 suited	18.62%	7.22	0.3448	0.3%	15.54%
A/3 suited	18.41%	7.26	0.336	0.3%	15.84%
K/T unsuited	18.18%	7.33	0.3328	0.9%	16.74%
K/8 suited	18.05%	7.33	0.3234	0.3%	17.04%
Pair of 7's	16.85%	7.77	0.309	0.45%	17.5%
Q/T unsuited	17.83%	7.32	0.3044	0.9%	18.4%
J/T unsuited	17.86%	7.3	0.3041	0.9%	19.31%
A/2 suited	17.86%	7.28	0.2997	0.3%	19.61%
T/8 suited	17.68%	7.32	0.2949	0.3%	19.91%
Q/8 suited	17.51%	7.34	0.285	0.3%	20.21%
J/8 suited	17.44%	7.33	0.2792	0.3%	20.51%
K/7 suited	17.57%	7.27	0.2777	0.3%	20.81%
9/8 suited	17.31%	7.38	0.2768	0.3%	21.12%
K/6 suited	17.17%	7.23	0.2413	0.3%	21.42%
Pair of 6's	15.84%	7.75	0.2282	0.45%	21.87%
K/5 suited	16.83%	7.2	0.2117	0.3%	22.17%
8/7 suited	16.38%	7.35	0.2033	0.3%	22.47%
K/4 suited	16.44%	7.22	0.1869	0.3%	22.78%
9/7 suited	16.2%	7.33	0.1868	0.3%	23.08%
T/7 suited	16.3%	7.26	0.1827	0.3%	23.38%
Q/7 suited	16.22%	7.25	0.1756	0.3%	23.68%
A/9 unsuited	16.27%	7.2	0.1711	0.9%	24.59%
J/7 suited	16.07%	7.26	0.1662	0.3%	24.89%
K/3 suited	16.06%	7.26	0.1653	0.3%	25.19%
Pair of 5's	14.93%	7.73	0.1542	0.45%	25.64%
7/6 suited	15.66%	7.33	0.1481	0.3%	25.94%
K/2 suited	15.7%	7.29	0.1446	0.3%	26.24%
Q/6 suited	15.85%	7.2	0.1417	0.3%	26.55%
T/9 unsuited	15.71%	7.21	0.1332	0.9%	27.45%
K/9 unsuited	15.62%	7.24	0.1308	0.9%	28.36%
8/6 suited	15.37%	7.3	0.1223	0.3%	28.66%
Q/5 suited	15.56%	7.17	0.1158	0.3%	28.96%
Pair of 4's	14.31%	7.76	0.1103	0.45%	29.41%
J/9 unsuited	15.34%	7.22	0.1081	0.9%	30.32%
6/5 suited	15.12%	7.32	0.1067	0.3%	30.62%
Q/9 unsuited	15.27%	7.23	0.1044	0.9%	31.52%
A/8 unsuited	15.55%	7.1	0.1037	0.9%	32.43%
Q/4 suited	15.16%	7.2	0.0916	0.3%	32.73%
9/6 suited	14.99%	7.26	0.0891	0.3%	33.03%
5/4 suited	14.74%	7.32	0.0789	0.3%	33.33%
T/6 suited	15.03%	7.18	0.0785	0.3%	33.63%
Pair of 3's	13.83%	7.8	0.0782	0.45%	34.09%
7/5 suited	14.74%	7.29	0.0741	0.3%	34.39%
Q/3 suited	14.81%	7.24	0.0721	0.3%	34.69%
J/6 suited	14.92%	7.17	0.0691	0.3%	34.99%
Pair of 2's	13.49%	7.83	0.057	0.45%	35.44%
Q/2 suited	14.46%	7.28	0.0524	0.3%	35.75%
A/5 unsuited	15.1%	6.95	0.0489	0.9%	36.65%
A/7 unsuited	14.94%	7.01	0.0473	0.9%	37.56%
J/5 suited	14.66%	7.13	0.0454	0.3%	37.86%
6/4 suited	14.07%	7.33	0.0306	0.3%	38.16%
8/5 suited	14.22%	7.24	0.0303	0.3%	38.46%
J/4 suited	14.28%	7.16	0.0222	0.3%	38.76%
A/4 unsuited	14.63%	6.96	0.0184	0.9%	39.67%
5/3 suited	13.71%	7.33	0.0049	0.3%	39.97%
J/3 suited	13.92%	7.2	0.0023	0.3%	40.27%
A/6 unsuited	14.39%	6.95	0	0.9%	41.18%
T/8 unsuited	14.07%	7.11	-0.0002	0.9%	42.08%
K/8 unsuited	14.01%	7.09	-0.0059	0.9%	42.99%
9/5 suited	13.81%	7.19	-0.0066	0.3%	43.29%
T/5 suited	13.98%	7.09	-0.0092	0.3%	43.59%
A/3 unsuited	14.15%	6.99	-0.0111	0.9%	44.49%
9/8 unsuited	13.73%	7.18	-0.0137	0.9%	45.4%
J/2 suited	13.59%	7.24	-0.0154	0.3%	45.7%
7/4 suited	13.48%	7.28	-0.0187	0.3%	46%
J/8 unsuited	13.68%	7.11	-0.0278	0.9%	46.91%
T/4 suited	13.64%	7.11	-0.0304	0.3%	47.21%
Q/8 unsuited	13.6%	7.11	-0.0331	0.9%	48.11%
4/3 suited	13.09%	7.37	-0.0351	0.3%	48.42%
T/3 suited	13.3%	7.15	-0.049	0.3%	48.72%
A/2 unsuited	13.54%	7.01	-0.0507	0.9%	49.62%
K/7 unsuited	13.48%	7	-0.0557	0.9%	50.53%
6/3 suited	12.85%	7.33	-0.0587	0.3%	50.83%
8/4 suited	12.98%	7.22	-0.0626	0.3%	51.13%
T/2 suited	12.95%	7.19	-0.0682	0.3%	51.43%
5/2 suited	12.5%	7.33	-0.0839	0.3%	51.73%
8/7 unsuited	12.79%	7.13	-0.0877	0.9%	52.64%
9/4 suited	12.71%	7.16	-0.0908	0.3%	52.94%
K/6 unsuited	13.02%	6.94	-0.0972	0.9%	53.85%
4/2 suited	12.08%	7.38	-0.1079	0.3%	54.15%
7/3 suited	12.25%	7.27	-0.1101	0.3%	54.45%
9/3 suited	12.37%	7.19	-0.1108	0.3%	54.75%
9/7 unsuited	12.54%	7.09	-0.1114	0.9%	55.66%
T/7 unsuited	12.55%	6.98	-0.1238	0.9%	56.56%
9/2 suited	12.03%	7.24	-0.1287	0.3%	56.86%
K/5 unsuited	12.65%	6.88	-0.1298	0.9%	57.77%
7/6 unsuited	12.08%	7.09	-0.1433	0.9%	58.67%
8/3 suited	11.88%	7.19	-0.1452	0.3%	58.97%
3/2 suited	11.49%	7.43	-0.1454	0.3%	59.28%
6/2 suited	11.62%	7.32	-0.1492	0.3%	59.58%
Q/7 unsuited	12.22%	6.95	-0.1511	0.9%	60.48%
J/7 unsuited	12.18%	6.97	-0.1514	0.9%	61.39%
K/4 unsuited	12.21%	6.9	-0.1579	0.9%	62.29%
8/2 suited	11.57%	7.23	-0.163	0.3%	62.59%
8/6 unsuited	11.7%	7.04	-0.1767	0.9%	63.5%
K/3 unsuited	11.79%	6.94	-0.1818	0.9%	64.4%
6/5 unsuited	11.51%	7.07	-0.1865	0.9%	65.31%
Q/6 unsuited	11.81%	6.87	-0.189	0.9%	66.21%
7/2 suited	11.19%	7.24	-0.1897	0.3%	66.52%
K/2 unsuited	11.41%	6.98	-0.2035	0.9%	67.42%
5/4 unsuited	11.12%	7.06	-0.2149	0.9%	68.33%
9/6 unsuited	11.22%	6.97	-0.2182	0.9%	69.23%
Q/5 unsuited	11.46%	6.82	-0.2191	0.9%	70.14%
7/5 unsuited	11.07%	7	-0.2249	0.9%	71.04%
T/6 unsuited	11.18%	6.83	-0.2361	0.9%	71.95%
Q/4 unsuited	11.03%	6.84	-0.2454	0.9%	72.85%
J/6 unsuited	10.94%	6.8	-0.2562	0.9%	73.76%
6/4 unsuited	10.39%	7.05	-0.2677	0.9%	74.66%
Q/3 unsuited	10.63%	6.88	-0.2679	0.9%	75.57%
8/5 unsuited	10.47%	6.92	-0.2757	0.9%	76.47%
J/5 unsuited	10.64%	6.73	-0.2837	0.9%	77.38%
Q/2 unsuited	10.26%	6.93	-0.289	0.9%	78.28%
5/3 unsuited	10.04%	7.04	-0.2936	0.9%	79.19%
J/4 unsuited	10.22%	6.76	-0.3091	0.9%	80.09%
9/5 unsuited	9.97%	6.82	-0.3206	0.9%	81%
7/4 unsuited	9.73%	6.95	-0.3238	0.9%	81.9%
T/5 unsuited	10.06%	6.65	-0.3308	0.9%	82.81%
J/3 unsuited	9.83%	6.81	-0.3312	0.9%	83.71%
4/3 unsuited	9.38%	7.08	-0.336	0.9%	84.62%
J/2 unsuited	9.46%	6.86	-0.3512	0.9%	85.52%
T/4 unsuited	9.66%	6.67	-0.3554	0.9%	86.43%
6/3 unsuited	9.08%	7	-0.3642	0.9%	87.33%
8/4 unsuited	9.12%	6.84	-0.3766	0.9%	88.24%
T/3 unsuited	9.28%	6.72	-0.3767	0.9%	89.14%
5/2 unsuited	8.73%	7	-0.3892	0.9%	90.05%
T/2 unsuited	8.93%	6.76	-0.3962	0.9%	90.95%
9/4 unsuited	8.76%	6.71	-0.4126	0.9%	91.86%
4/2 unsuited	8.3%	7.06	-0.4138	0.9%	92.76%
7/3 unsuited	8.41%	6.88	-0.4219	0.9%	93.67%
9/3 unsuited	8.4%	6.74	-0.4338	0.9%	94.57%
9/2 unsuited	8.04%	6.8	-0.4531	0.9%	95.48%
3/2 unsuited	7.67%	7.12	-0.4543	0.9%	96.38%
6/2 unsuited	7.79%	6.94	-0.46	0.9%	97.29%
8/3 unsuited	7.96%	6.73	-0.4646	0.9%	98.19%
8/2 unsuited	7.61%	6.77	-0.4844	0.9%	99.1%
7/2 unsuited	7.28%	6.77	-0.5073	0.9%	100%
Total	7.2	0	100%

Methodology

This table is the result of a random simulation of 25,729,704,000 games and assumes all players stay in until the end of the hand.

The following table shows my power rating for each initial 2-card hand in a 8-player game. The numbers are on a 0 to 40 scale. Basically, you should only play hands that are dark green, blue, or purple. Of course you should be more be more liberal in late position and picky in early position.

Use the top table if you have a pair, the middle table if your cards are suited, and the bottom table if your cards are unsuited. Except for a pair,look up your high card along the left and your low card along the top.

Inside Links

Written by: Michael Shackleford